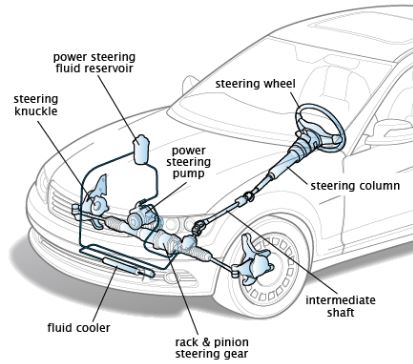
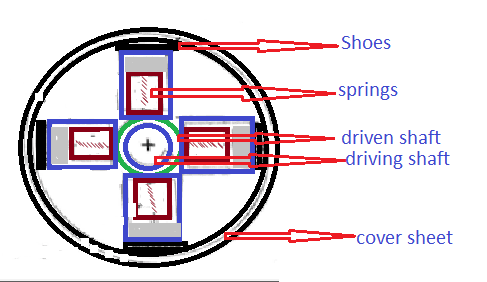
Bukingham-π(pi) Theorem
This Bukingham-π(pi) theorem is used to determined the relation by discribing the physical phenomenon to the number of independent dimensions groups.
The non dimension group is given by that a large number of physical variable are involved, in a dimensionally homogeneous containing ‘m’ fundamental dimensions. It is given by (n-m).
The equation is given by the relation amount the variables.
F(π1,π2,π3,π4………..)
For example, if there are 8 variables ins problem and this is expressed in 6 terms, then by using the buckingham-π theorem the dimensionless groups are represented as 8-6=2. This is expressed as
F(π1,π2).